แรงดันหัวฉีด, เส้นผ่านศูนย์กลางรูหัวฉีด และอัตราการไหล

อัตราการไหลของหัวฉีดเกี่ยวข้องอย่างใกล้ชิดกับขนาดรูหัวฉีดและแรงดัน การเพิ่มแรงดันหรือขนาดรูหัวฉีดจะทำให้อัตราการไหลสูงขึ้น แต่การเพิ่มนี้ไม่ใช่เชิงเส้น ในความเป็นจริง ภายใต้แรงดันเดียวกัน อัตราการไหลจะแปรผันตามกำลังสองของเส้นผ่านศูนย์กลางรูหัวฉีด ในทำนองเดียวกัน เมื่อขนาดรูหัวฉีดคงที่ อัตราการไหลจะแปรผันตามรากที่สองของแรงดัน

สำหรับรุ่นหัวฉีดเดียวกันจาก LORRIC มีตัวเลือกอัตราการไหลหลากหลาย ซึ่งสามารถทำได้โดยการออกแบบขนาดรูหัวฉีดที่แตกต่างกันและจับคู่กับช่วงแรงดันที่เหมาะสม กล่าวอีกนัยหนึ่ง ลูกค้าสามารถเลือกสเปคหัวฉีดที่เหมาะสมได้โดยอ้างอิงถึงรหัสอัตราการไหลและแรงดันเพื่อให้ตรงตามความต้องการ

บทความนี้จะนำผู้อ่านทำความเข้าใจความสัมพันธ์ระหว่างอัตราการไหล แรงดัน และขนาดรูหัวฉีด

เมื่อแรงดันเพิ่มขึ้น อัตราการไหลจะเพิ่มขึ้นตามรากที่สองของแรงดัน แต่ทำไมถึงเป็นเช่นนั้น? อัตราการไหลของหัวฉีดถูกกำหนดโดยสูตรดังต่อไปนี้:

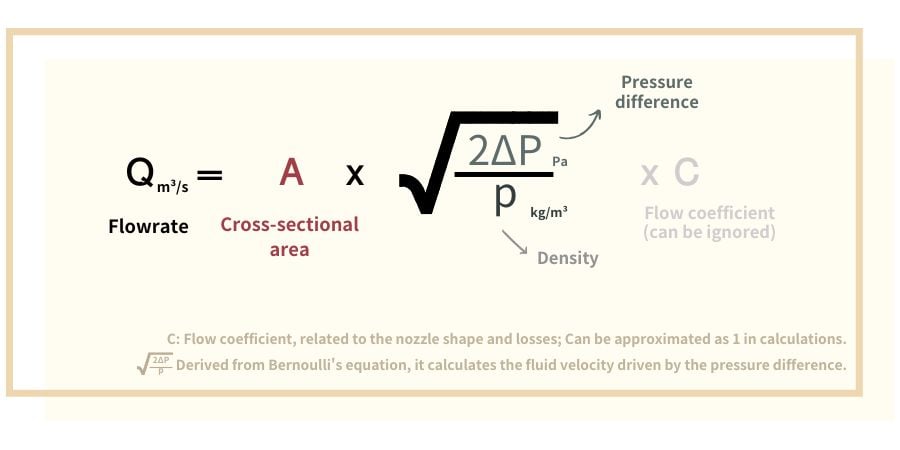
• ดังนั้นอัตราการไหลเปลี่ยนแปลงอย่างไรเมื่อแรงดันเพิ่มขึ้น?
จากสูตรข้างต้น เราสามารถเห็นได้ว่า:
เมื่อแรงดันเพิ่มขึ้น อัตราการไหลก็เพิ่มขึ้นเช่นกัน แต่ไม่เป็นเส้นตรง แทนที่จะเป็นความสัมพันธ์แบบรากที่สอง
ถ้าแรงดันเพิ่มขึ้นจาก 2 บาร์เป็น 8 บาร์ (4 เท่า) อัตราการไหลจะเพิ่มขึ้นเพียง √4 = 2 เท่า
กล่าวคือ: อัตราการไหล Q แปรผันตามรากที่สองของความแตกต่างของแรงดัน ΔP
ดังนั้นเราสามารถอธิบายความสัมพันธ์ระหว่างอัตราการไหล Q และแรงดัน P ได้ดังนี้:
ด้วยวิธีนี้ เราสามารถใช้ความสัมพันธ์ระหว่างอัตราการไหลและความแตกต่างของแรงดันในการคำนวณค่าได้อย่างง่ายดาย ตัวอย่างเช่น เมื่อเปลี่ยนเงื่อนไขการทำงานของหัวฉีด (เช่น อัตราการไหล) สูตรนี้สามารถใช้เพื่อกำหนดการเปลี่ยนแปลงแรงดันที่ต้องการ หรือในทางกลับกัน เพื่อหาค่าอัตราการไหลในแรงดันที่ต่างกัน
...